AEROFOIL THEORY

Every theory will contain some assumptions and some basic tools required.Here those basic tools will be discussed.

>>Primarily consider the surface of the aerofoil is with vortex completely and this is applicable for
only thin aerofoil.

>>FOr thin aerofoil to formulate flow characteristics over the aerofoil are obtained as follows:

 

     *On the surface of the aerofoil i.e upper and lower surfaces,simple vortex is assumed everywhere.

     *If aerofoil is viewed from for away distance it appears to fall on chord  or chamberline.

     *Hence is assumed that to analysing flow characteristics over aerofoil simple vortex on the chord
or chamberline.

BASIC TOOLS:

>> Consider a simple vortex at point "o" with strength  Г .

>> Imagie a straight line passing through origin "o".

      and the straight line is etended to the -∞ to +∞ .

 >>Infinite number of simple vortex on the straight line to be imagined.

>>Call the line as the straight vortex filament and strength of the vortex filament is  Г as the simple
vortex.

>>The straight vortex filament induces a velocity and imagine infinite number of straight vorte filaments.

>>The side by side vortex filaments also forms straight vortex sheet. 

>>The strength of each straight vortex filament is very small.

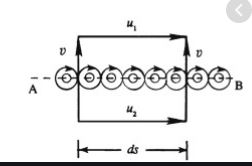
>>Consider the edge view of the vortex sheet we obtain a curve line in XZ-plane.

>>Since it is a continues portion of the sheet taking up the distance measured from a to b is S.

>>Consider a small distance ds. ४ is the strength of the vortex line sheet per unit length.

           strength of the vortex sheet of length   ds=४.ds

>>The strength of entire vortex sheet=Г.

                                  

>>Consider a point P in the element distance 'r'. r is the distance from the point p to the element vortex sheet.

>>The velocity induced at elemental vortex sheet will be as dv is the velocity induced by elemental vortex sheet.

                                                           dv=(-४.ds)/2πr.

>>Consider elemental size of vortex sheet of ds.Length in a rectangle box.

>>Circulation around the rectangular box or closed path.

४=(u1-u2)

>>It states that local jump in tangential velocity across the vortex sheet equal to the local strength of the sheet with the given length.

KUTTA condition:

>>Actual airfoil trailing edge is having a finite angle.In theoritical aerofoil the upper surface and lower surface will form a point.

>>Velocity along the upper surface and lower surface is v1 and v2. v1 is parallel to upper surface at point a. v2 is parallel to lower surface at point a.

>>For the finite angle trailing edge it appears that at point a. we will have two different velocities in two different direction.

>>It is not physically possible if v1 and v2 are finite.only possible when v1=v2=0.

>>For the finite angle trailing edge point a trailing edge is a stagnation point.

>>For theoritical aerofoil v1=v2=0.since direction is same.

summary:

>>For a given aerofoil at a give angle of attack the value of  ४  around aerofoil is such that the flow leaves the trailing edge smoothly.

>>If the trailing edge is a finite angle,trailing edge is the stagnation point.

>>If the trailing edge is the cuspid then the velocities leaving the top and bottom surface are finite and equal in magnitude and direction.